Surface brightness

The overall brightness of an extended astronomical object such as a galaxy, star cluster, or nebula, can be measured by its total magnitude, integrated magnitude or integrated visual magnitude; a related concept is surface brightness, which specifies the brightness of a standard-sized piece of an extended object.

Contents

General description

The total magnitude is a measure of the brightness of an extended object such as a nebula, cluster or galaxy. It can be obtained by summing up the luminosity over the area of the object. Alternatively, a photometer can be used by applying apertures or slits of different sizes diameter.[1] The background light is then subtracted from the measurement to obtain the total brightness.[2] The resulting magnitude value is the same as a point-like source that is emitting the same amount of energy.[3]

The apparent magnitude of an astronomical object is generally given as an integrated value—if a galaxy is quoted as having a magnitude of 12.5, it means we see the same total amount of light from the galaxy as we would from a star with magnitude 12.5. However, while a star is so small it is effectively a point source in most observations (the largest angular diameter, that of R Doradus, is 0.057 ± 0.005 arcsec), the galaxy may extend over several arcseconds or arcminutes. Therefore, the galaxy will be harder to see than the star against the airglow background light. Quoting an object's surface brightness gives an indication of how easily observable it is.

Calculating surface brightness

Surface brightnesses are usually quoted in magnitudes per square arcsecond. Because the magnitude is logarithmic, calculating surface brightness cannot be done by simple division of magnitude by area. Instead, for a source with magnitude m extending over an area of A square arcseconds, the surface brightness S is given by

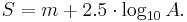
S = m %2B 2.5 \cdot \log_{10} A.

Surface brightness is constant with luminosity distance. For nearby objects, the luminosity distance is equal to the physical distance of the object. For a nearby object emitting a given amount of light, radiative flux decreases with the square of the distance to the object, but the physical area corresponding to a given solid angle (e. g. 1 square arcsecond) increases in the same fashion, resulting in the same surface brightness.[4]

Relationship to physical units

The surface brightness in magnitude units is related to the surface brightness in physical units of solar luminosities per square parsec by

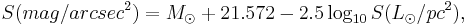
S(mag/arcsec^2)=M_{\odot}%2B21.572-2.5\log_{10} S (L_{\odot}/pc^2),

where 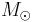M_{\odot} and L_{\odot} are the absolute magnitude and the luminosity of the Sun in chosen color-band[5] respectively.

See also

References

  1. ^ Daintith, John; Gould, William (2006). The Facts on File dictionary of astronomy. Facts on File science library (5th ed.). Infobase Publishing. p. 489. ISBN 0816059985. 
  2. ^ Palei, A. B. (August 1968). "Integrating Photometers". Soviet Astronomy 12: 164. Bibcode 1968SvA....12..164P. 
  3. ^ Sherrod, P. Clay; Koed, Thomas L. (2003). A Complete Manual of Amateur Astronomy: Tools and Techniques for Astronomical Observations. Astronomy Series. Courier Dover Publications. p. 266. ISBN 0486428206. 
  4. ^ Sparke & Gallagher (2000, § 5.1.2)
  5. ^ Absolute magnitudes of the Sun in different color-bands can be obtained from Binney & Merrifield (1998) or Absolute Magnitude of the Sun in Several Bands

General references